|
(a)Use De Morgan ’s laws to write negations for the following
statements.
(i)Chee Leong is a multimedia major and Chee Leong ’s sister
is a computer science major.[2 marks ]
(ii)The power is switched off or the machine is unplugged.[2 marks
]
(i)Chee Leong is not a multimedia major
or Chee Leong ’s sister is not a computer science major.One mark
for or and one mark for both nots. [2 marks ]
(ii)The power is not switched off and the machine is not unplugged.One
mark for and and one mark for both nots. [2 marks ]
(b)Construct truth tables for the following statements.
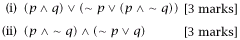
Which statement is a tautology and which is a contradiction?Justify
your
answer.[2 marks ]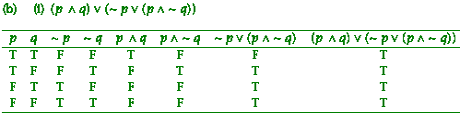
Three marks. Subtract one mark for each error up to a maximum of
three. [3 marks ]
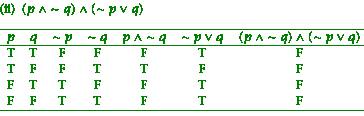
Three marks. Subtract one mark for each error up to a maximum of
three. [3 marks ]
(i)is a tautology as it is always true.One mark. (ii)is a contradiction
because it is
always false.One mark. Do not award any marks unless both the correct
answer and reason are given. [2 marks ]
(c)Disprove the following statements by giving a counterexample.
(i)The product of any two prime numbers is prime.[1 mark ]
(ii)The difference of any two odd integers is odd.[1 mark ]
(iii)For all integers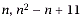 
is a prime number.[1 mark ]
Award marks for
any suitable contradiction.
(i)E.g.3 and 5 are both prime numbers,but 3 ×5 =15 is not prime.[1
mark ]
(ii)E.g.5 and 3 are odd integers,but 5 -3 =2 is even.[1 mark ]
(iii)E.g.When n =11,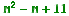
=121 is not prime.[1 mark ]
|